Q51261 A car company is faced with an allocation problem resulting from a rental agreement, which allow cars to be returned to locations other than those from where they were originally rented. At the present time there are two locations with 15 and 13 cars respectively and 4 locations requiring 9, 6, 7 and 9 cars respectively. Table 1 depicts the unit transportation costs (in dollars) between the locations.

Destinations

		D1	D2	D3	D4
Sources	S1	45	17	21	30
	S2	14	18	19	31

Table 1 Unit Transportation Costs between Locations

Obtain a minimum cost schedule.

Solution:

Since the supply and requirements are not equal it is called an unbalanced transportation problem. In general, if £a_i ≠ £b_j then it is called an unbalanced transportation problem. We introduce either a dummy row or a column with cost zero quantities and £b_i ≠ £a_j respectively. Applying Vogel’s approximation method we find the basic feasible solution. Table 2 depicts the basic feasible solution.

Table 2: Basic Feasible Solution using Vogel’s Approximation Method

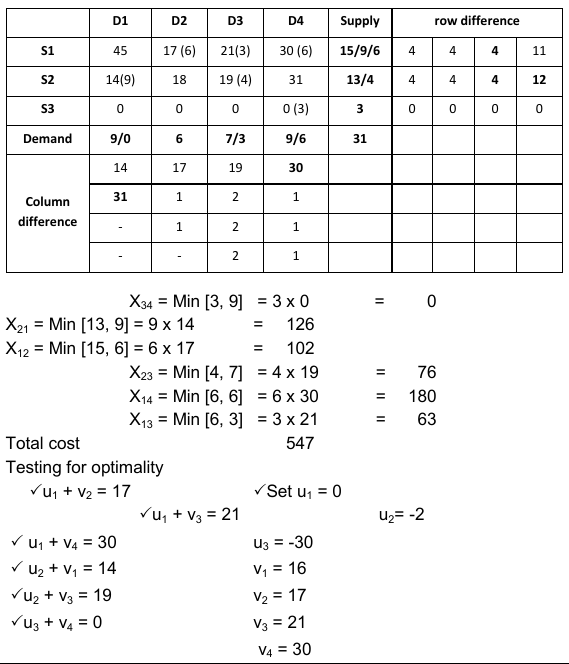
For non-allocated cells, determine c_ij– u_I– v_i. Since all the quantities are non-negative, the current solution is optimal. The minimum transportation cost is equal to:

6×17+3×21+6×30+9×14+4×19+3×0 = 547.

This is achieved by transporting x₁₂ = 6 cars from source 1 to destination 2, x₁₃ = 3, x₁₄ = 6 cars from sources 1 to destinations 3 and 4 respectively; x₂₁ = 9 and x₃₃ = 4 cars from sources 2 to destinations 1 and 3 respectively.

DistPub Team

Distance Publisher (DistPub.com) provide project writing help from year 2007 and provide writing and editing help to hundreds student every year.