Q51198a Hungarian Approach ; Let Ui denote the ith row constant which is added to ith row elements and Vj denote the jth column constant which is added to elements of jth column.

I II III IV Ui
A 10 12 19 11 U1 = 10
B 5 10 7 8 U2 = 5
C 12 14 13 11 U3 = 8
D 8 15 11 9 U4 = 2
Vj V1 = 5 V2 = 8 V3 = 7 V4 = 9

Total Ui = SUi = 25

Total Vj = SVj = 29

Solution

21 8 image 826
21 8 image 827

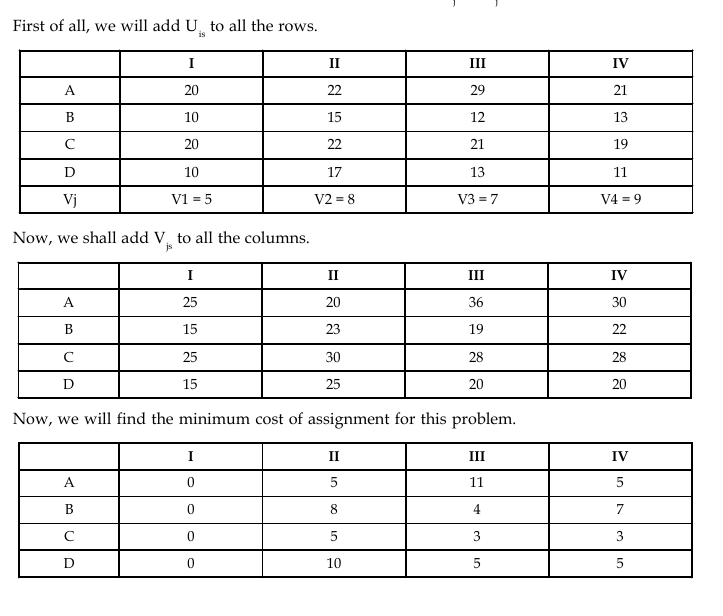
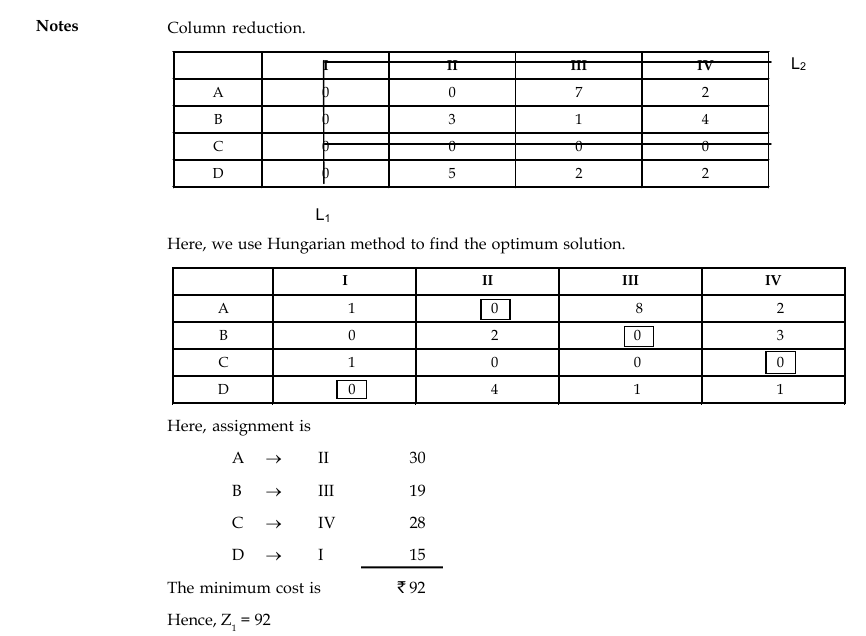
We got the cost matrix for Z1 by adding U1 to different rows and Vj  to different columns of cost matrix for Z.

Hence, Z1 = Z + SUi + SVj

i.e.,     92 = 38 + 25 + 29

Observe that the assignment for both the matrix is same. Hence, we can say that Z is minimized whenever Z1 is minimized. However, the solution (minimised cost for Z = and Z1) will be different due to cost elements (Cij).

ed010d383e1f191bdb025d5985cc03fc?s=120&d=mm&r=g

DistPub Team

Distance Publisher (DistPub.com) provide project writing help from year 2007 and provide writing and editing help to hundreds student every year.